

Validation of a new model-based tracking technique for measuring three-dimensional, in vivo glenohumeral joint kinematics. A comparison of the accuracy of several hip center location prediction methods. Effects of movement for estimating the hip joint centre.
#POSTVIEW PRINCIPAL CURVATURE SKIN#
However, the improvements observed when using the DF-based solutions suggest that skin motion artifact is a large source of error for the functional methods.īegon, M., T. Overall, functional methods were superior to predictive methods for HJC estimation.
The Harrington method was the best predictive technique using both skin markers (13.2 ± 6.5 mm) and DF-based solutions (10.6 ± 2.5 mm). Using skin markers, functional methods had better mean agreement with the HJC measured by DF (11.0 ± 3.3 mm) than predictive methods (18.1 ± 9.5 mm) estimates from functional and predictive methods improved when using the DF-based solutions (1.3 ± 0.9 and 17.5 ± 8.6 mm, respectively). Additionally, DF-based solutions generated virtual markers placed on bony landmarks, which were input to the predictive and functional methods to determine if estimates of the HJC improved. Eleven non-pathologic subjects were imaged with DF and reflective skin marker motion capture.

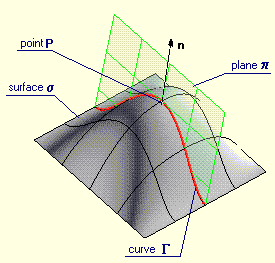
Using dual fluoroscopy as the reference standard, we then assessed the accuracy of three predictive and two functional methods. In this study, we used dual fluoroscopy (DF) (a dynamic X-ray imaging technique) and three-dimensional reconstructions from computed tomography images, to measure HJC locations in vivo. Quantifying the accuracy of these methods is an area of open investigation. Skin motion artifact may introduce errors in the estimate of HJC for both techniques. Here, predictive techniques use regression equations of pelvic anatomy to estimate the HJC, whereas functional methods track motion of markers placed at the pelvis and femur during a coordinated motion. Most often, the hip joint center (HJC) is derived from locations of reflective markers adhered to the skin. Things look very different in higher dimensions.Predictions from biomechanical models of gait may be sensitive to joint center locations. In an appropriate identification that number coincides with the determinant of the Weingarten map, i.e. However, in two dimensions, the space of such maps is one-dimensional, so again you can reduce it to one number. The Riemann cuvature in general is a much more complicated object (a trilinear map from three copies of the tangent space to the tangent space with certain symmetries). These are easily seen to coincide with the two eigenvalues of the Weingarten map and thus with the principal cuvatures. As such, it is a smooth function on the unit circle, which (if non-constant) has a unique minumum and a unique maximum. The usual interpretation of the normal cuvature is as the restriction of the quadratic form defined by this symmetric bilinear form to the unit sphere in the tangent space. Using the inner product, the Weingarten map can be converted to a symmetric bilinear form (the second fundamental form) on the tangent space. The principal curvatures (which are indeed numbers) are the eigenvalues of this linear map. In the setting of surfaces, I would say that the basic curvature object is the Weingarten map, which, in a point, is a symmetric endomorphism of the tangent plane at that point.

One often reduces to numbers in the end (since these are easy to compare), but intially, one has more general objects. However, both in classcial differential geometry and in Riemannian geometry, a "curvature" is not necessarily a number. You arre right in principle that curvature is attached to a point.


 0 kommentar(er)
0 kommentar(er)
